Обратное преобразования Лапласа онлайн, калькулятор для оригиналов функций по изображению.
Теория функций комплексного переменного.
: x^a
модуль x: abs(x)
: Sqrt[x]
: x^(1/n)
: a^x
: Log[a, x]
: Log[x]
: cos[x] или Cos[x]
 : sin[x] или Sin[x]
: sin[x] или Sin[x] : tan[x] или Tan[x]
: tan[x] или Tan[x] : cot[x] или Cot[x]
: cot[x] или Cot[x] : sec[x] или Sec[x]
: sec[x] или Sec[x] : csc[x] или Csc[x]
: csc[x] или Csc[x] : ArcCos[x]
: ArcCos[x]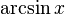 : ArcSin[x]
: ArcSin[x] : ArcTan[x]
: ArcTan[x] : ArcCot[x]
: ArcCot[x] : ArcSec[x]
: ArcSec[x]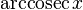 : ArcCsc[x]
: ArcCsc[x] : cosh[x] или Cosh[x]
: cosh[x] или Cosh[x] : sinh[x] или Sinh[x]
: sinh[x] или Sinh[x] : tanh[x] или Tanh[x]
: tanh[x] или Tanh[x] : coth[x] или Coth[x]
: coth[x] или Coth[x] : sech[x] или Sech[x]
: sech[x] или Sech[x] : csch[x] или Csch[е]
: csch[x] или Csch[е] : ArcCosh[x]
: ArcCosh[x] : ArcSinh[x]
: ArcSinh[x] : ArcTanh[x]
: ArcTanh[x]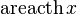 : ArcCoth[x]
: ArcCoth[x] : ArcSech[x]
: ArcSech[x] : ArcCsch[x]
: ArcCsch[x]
Преобразованием Лапласа некоторой функции
называется интегральное преобразование вида:
Функция
называется оригиналом, функция
— изображением. Причём
является функцией комлексной переменной, т.е.
.
В качестве примера, найдём изображение
функции оригинала
.
Для этого нам необходимо воспользоваться приведённой выше формулой и
вычислить интеграл:
То, что функция
является изображением функции
записывается как
или
.
Важным свойством
преобразования Лапласа
является то, что если
, то
Указанное свойство активно используется при
решении дифференциальных уравнений
поскольку позволяет сводить последние к алгебраическим.
Наш онлайн калькулятор, построенный на основе системы Wolfram Alpha позволяет найти преобразование Лапласа практически любой, даже очень сложной функции.
Интегральное преобразование Лапласа онлайн, калькулятор изображения функций.
Теория функций комплексного переменного.
: x^a
модуль x: abs(x)
: Sqrt[x]
: x^(1/n)
: a^x
: Log[a, x]
: Log[x]
: cos[x] или Cos[x]
 : sin[x] или Sin[x]
: sin[x] или Sin[x] : tan[x] или Tan[x]
: tan[x] или Tan[x] : cot[x] или Cot[x]
: cot[x] или Cot[x] : sec[x] или Sec[x]
: sec[x] или Sec[x] : csc[x] или Csc[x]
: csc[x] или Csc[x] : ArcCos[x]
: ArcCos[x]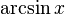 : ArcSin[x]
: ArcSin[x] : ArcTan[x]
: ArcTan[x] : ArcCot[x]
: ArcCot[x] : ArcSec[x]
: ArcSec[x]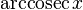 : ArcCsc[x]
: ArcCsc[x] : cosh[x] или Cosh[x]
: cosh[x] или Cosh[x] : sinh[x] или Sinh[x]
: sinh[x] или Sinh[x] : tanh[x] или Tanh[x]
: tanh[x] или Tanh[x] : coth[x] или Coth[x]
: coth[x] или Coth[x] : sech[x] или Sech[x]
: sech[x] или Sech[x] : csch[x] или Csch[е]
: csch[x] или Csch[е] : ArcCosh[x]
: ArcCosh[x] : ArcSinh[x]
: ArcSinh[x] : ArcTanh[x]
: ArcTanh[x]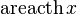 : ArcCoth[x]
: ArcCoth[x] : ArcSech[x]
: ArcSech[x] : ArcCsch[x]
: ArcCsch[x]Subscribe to verify your answer
Subscribe
Sign in to save notes
Sign in
Number Line
Examples
-
laplace:e^{frac{t}{2}}
-
laplace:e^{-2t}sin^{2}(t)
-
laplace:8pi
-
laplace:g(t)=3sinh(2t)+3sin(2t)
-
inverse:laplace:frac{s}{s^{2}+4s+5}
-
inverse:laplace:frac{1}{x^{frac{3}{2}}}
-
inverse:laplace:frac{sqrt{pi}}{3x^{frac{3}{2}}}
-
inverse:laplace:frac{5}{4x^2+1}+frac{3}{x^3}-5frac{3}{2x}
- Show More
Description
Find the Laplace and inverse Laplace transforms of functions step-by-step
Frequently Asked Questions (FAQ)
-
How do you calculate the Laplace transform of a function?
- The Laplace transform of a function f(t) is given by: L(f(t)) = F(s) = ∫(f(t)e^-st)dt, where F(s) is the Laplace transform of f(t), s is the complex frequency variable, and t is the independent variable.
-
What is mean by Laplace equation?
- The Laplace equation is a second-order partial differential equation that describes the distribution of a scalar quantity in a two-dimensional or three-dimensional space. The Laplace equation is given by: ∇^2u(x,y,z) = 0, where u(x,y,z) is the scalar function and ∇^2 is the Laplace operator.
-
What kind of math is Laplace?
- Laplace transforms are a type of mathematical operation that is used to transform a function from the time domain to the frequency domain. They are a specific example of a class of mathematical operations called integral transforms.
-
Why is it called Laplace?
- The Laplace equation is named after the discoverer Pierre-Simon Laplace, a French mathematician and physicist who made significant contributions to the field of mathematics and physics in the 18th and 19th centuries.
-
What does the Laplace equation use for?
- The Laplace equations are used to describe the steady-state conduction heat transfer without any heat sources or sinks
- Show more
laplace-calculator
en
Related Symbolab blog posts
Practice Makes Perfect
Learning math takes practice, lots of practice. Just like running, it takes practice and dedication. If you want…
Read More
|
19:26 найти оригинал функции по ее изображению |
|
Калькулятор для оригиналов функций по изображению (калькулятор обратного преобразования Лапласа ) |
Категория: Теория функций комплексного переменного | Просмотров: 34918 | Добавил: Admin | Теги: тфкп | Рейтинг: 4.5/2 |


 : x^a
: x^a : Sqrt[x]
: Sqrt[x]![sqrt[n]{x}](https://upload.wikimedia.org/math/5/e/4/5e4352778f3b156f05ef056f9793ec36.png) : x^(1/n)
: x^(1/n) : a^x
: a^x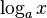 : Log[a, x]
: Log[a, x]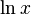 : Log[x]
: Log[x] : cos[x] или Cos[x]
: cos[x] или Cos[x]